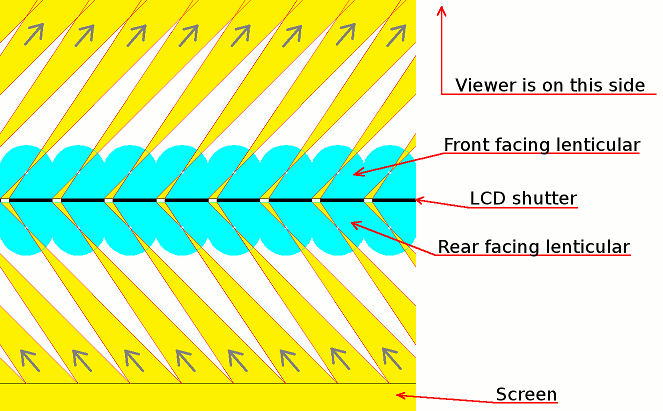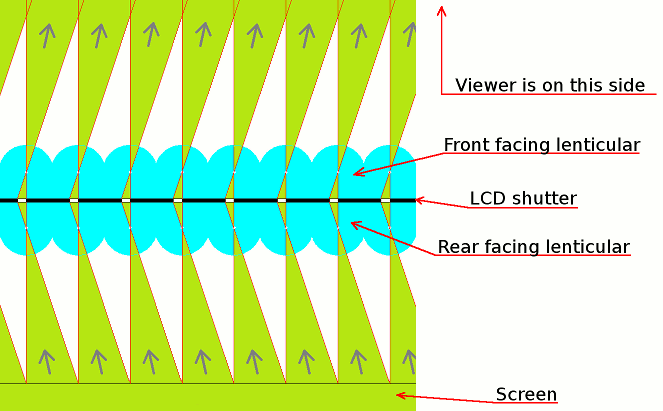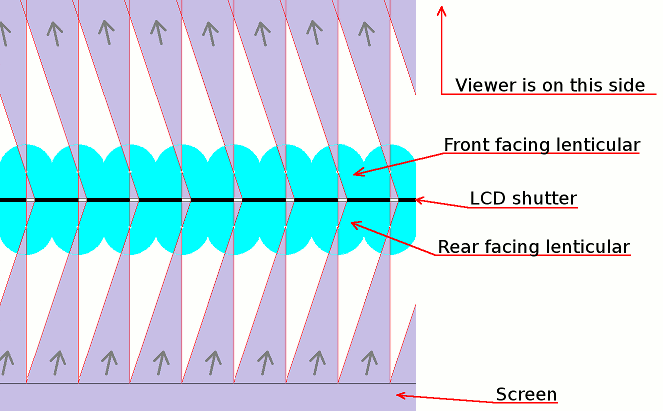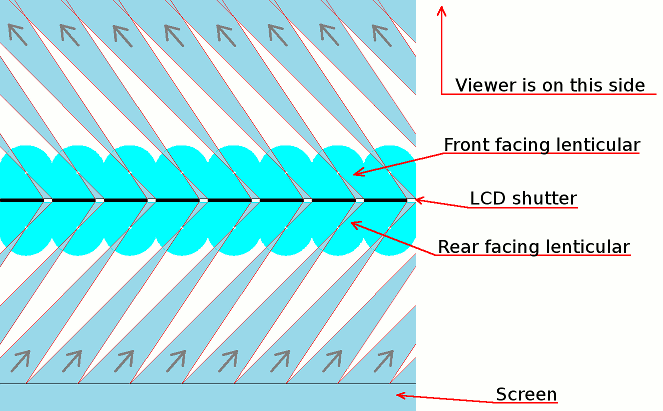
A dual lenticular lens is a pair of lenticulars with the same pitch put flat sides together and with lenses on the reliefs. Optically, each pair of the symmetric lenses forms a cylindrical analogue of the Cutrona cell scheme with spherical lenses. In engineering practice, this scheme is used as an nondistortive joint for fiber segments (optical fiber). It is obvious that a cross section of the cylindrical pair is identical to any axial section of Cutrona scheme, i.e. the optical properties of the scheme corresponding to the axial cross-section (conjugation, tautohronism) are fully inherited also by the cylindrical lens pair in a dual lenticular lens. Then one can definitely assume that any symmetric cylindrical pair of lenses of the dual lenticular lens makes no distortions (maintaining the amplitude and phase) for an incoming light stream, orthogonal to the cylindrical lens surface. Furthermore, a common focal plane of the cylindrical pair, similarly as in the Cutrona scheme, is a Fourier plane, i.e. an area of Fourier convolution of an incoming light flow, and therefore it is the best location to place translucent layers for controlling light flow and image reconstruction (Ernst Abbe). Therefore, the constructive purpose of a dual lenticular lens is to contain a special translucent layer within, right on the way of a light flow (here, i.e. in SSE – the active slit layer and scattering layer).
An active slit layer is an inner layer between the dual lenticular lens, which is located on the way of a light flow coming from a screen. The slits are arranged in parallel to the lenses with the same pitch, and the transverse dimension of each slit is equal to the strip width (i.e., to the compressed perspective) on the standard Lippmann-Bonnet image. In other words, only one slit out of several possible located under each lens. All synchronous open slits occupy the same position on the Lippmann-Bonnet image. Additionally the switching of slits is synchronized with change of frames on a projection screen (image). Moreover, all the slits are switched simultaneously and in the same direction, i.e. exactly by one slit position. Thus, each switch cycle corresponds to one of scene angles, and each slit located under the corresponding lens sequentially scans all perspective strips on the Lippmann-Bonnet image. In the next cycle, the slits are positioned for the next scene angle, etc.
Object-based optics of SSE includes an image on the projection screen, an inner lenticular lens and a slit layer. The relative positions of these components and optical interaction in the form of a fragmented projective (compressive) display are seen in the following illustration:
The illustration shows that each lens “cuts” a portion of image displayed on the projection screen and projects it in compressed form in accordance with the size of the slit, i.e., the fragmentary compression of the image on the screen comes without any pixel loss, and therefore unresolvable defects are avoided. Thus, all fragments have identical linear dimensions, corresponding to lenticular pitch, i.e., they do not overlap and any non-projected intervals do not occur between them. The illustration also shows that with the transition (shift) of the slit layer from the moment t to the moment t + 1 (performed simultaneously with the change of a frame on the screen) all projected features of the optical scheme remain preserved, i. e. a new image on the demonstration screen just turns into a compressed fragmented image without any losses in new slit positions. In fact, the given explanations of ability to project each perspective imply the possibility of replacing an inner lenticular lens with a fixed optically equivalent slit layer (potential to reduce costs of the system).
The image below shows a tip of an ordinary office pen, placed under a lenticular lens, which is photographed from the flat side of a lenticular lens:
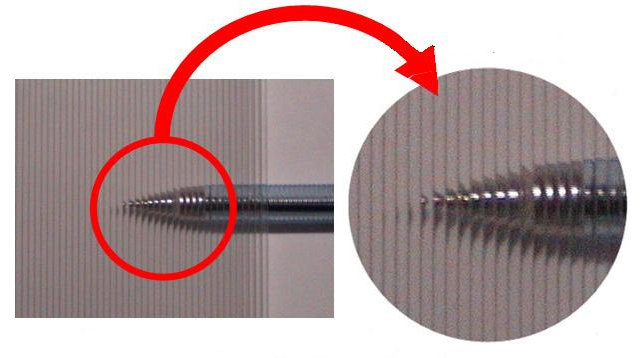
A fixed lens camera thus captures the view onto an array of slits from a specific position. You can see compressed and inverted (horizontally) “stripped” presentations (Fourier images) of a real image of a pen that form under each lens – exactly as is required for correct Lippmann-Bonnet chart. In other words, a pair comprised of a lenticular lens and a slit layer (i.e., a fixed set of slits) without any constructive additions (and special processors) is able to synthesize the appropriate set of “stripes” of one perspective needed for a correct Lippmann-Bonnet image. It is obvious that with change of the frame/perspective on the screen (i.e., when the next scene perspective is demonstrated), the slits move synchronously to the next (adjacent) position, and similarly form a “stripped set” of the new perspectives in the new/next slit layer (through the lenticular lens) on the Lippmann-Bonnet chart. Thus, the dynamic Lippmann-Bonnet image scheme is formed during the ordinary course of demonstration of frames on the screen provided that the organization of video sequence, (mentioned earlier in this section) follows the scheme for N perspective synthesis. The difference between the dynamic and the classical static image is that in the static image all “stripped” images of different angles appear at the same time (computer synthesis) and in the dynamic version they occur one by one, instantly and sequentially, corresponding to the ordered change of screen frames. I.e., in the dynamic Lippmann-Bonnet image, a compressed image corresponding a particular angle is presented at any moment in time and the remaining positions remain dark, as they are closed by opaque areas of the slit layer. In principle, this optical scheme implements the same shutter as in some 3d glasses, but only for more than 2 angles, and no glasses are required. For further clarification it should be noted that software-based full Lippmann-Bonnet image generation may come with a delay due to processing and is accompanied by unavoidable distortions of digitally compressed perspectives (due to pixel loss), but the dynamic version allows to avoid them, because everything happens instantly .
Of course, with the increasing number of perspectives increases the distortion from digital compression to the Lippmann-Bonnet image. Having in mind proportionality of this relationship (only for horizontal parallax!) and starting with a certain threshold value of this number (directly based on viewers’ 3D visual quality assessment), discrete image forming method becomes unacceptable. Each subsequent step increasing resolution of a LCD screen only slightly improves the result either by a large number of sub-perspective pixels count(i. e. partial enhancement of the 3D-image perspective quality ), or by small addition of new perspectives of the former quality. However, we emphasize it again, the defects of discrete compression are unavoidable in principal and, consequently, the threshold of critical quantity of perspectives number is low, for the same basic reasons…
The situation is different using a dynamic Lippmann-Bonnet chart. Here, every angle is rendered in the original quality, i. e. ideally without any visual defects caused by loss of pixels. At the same time, the number of possible angles is limited only by screen refresh rate (in combination with the existing synchronous slit layer) and by screen brightness reserve. Modern screens with a new generation of liquid crystals have massively exceeded 200-240 Hz refresh rate, and an equally strong LED back light significantly strengthened their brightness. Some screen models feature up to 800 Hz refresh rate. With sufficient demand of 50 Hz for each angle (needed to avoid the visual presence of “dark flickering”) and with actual reserve of brightness, it means no less than 8-16 angle volumetric image in a simple optical system with a simple video streamimg algorithm, and without procedural delays in synthesis of the Lippmann-Bonnet image. We also emphasize that developers of the new-generation LCD screens have already announced conceptual models with up to 7 kHz refresh rate. This means that even with an excessive reserve on the frequency part, and with increase in the specific frequency range for one angle (up to 65 Hz – a threshold value of critical frequency when dark flickering becomes visible according to Ferry-Porter’s law), the dynamic version of the Lippmann-Bonnet image will enable simple rendering of more than 50 high quality angles for 3D scene using consumer-level technology (this is very important for the industrial development of SSE).
Let us return to the device structure. SSE consists of two mutually complementary parts: The first part is a lenticular lens facing a projection screen paired with an active slit layer, and the second part is a scattering surface and a lenticular lens facing the audience. Together this “sandwich” forms a dual lenticular lens with embedded optical layers as was shown in the illustration at the beginning of this section. The first part, i. e. the active part of the dual lenticular lens, creates the dynamic Lippmann-Bonnet image, and the second, i. e. the passive part of the dual lenticular lens, directs optical flow of the perspectives consecutively appearing on the screen to the audience area in compliance with a predetermined parallax. In other words, with the proper organization of incoming video sequence, at the output, i. e., in the audience zone will appear normally visible volume (3D) effect just like in the classical lenticular cards (by virtue of the earlier mentioned Talbot law). Above in the text it has already been noted that, in view of projective action, an inner lenticular lens (facing the projection screen) of a dual lenticular lens may be substituted with a passive (i. e. static) slit layer with thin vertical slits, which play the role of optical centres of the cylindrical lenses. For the same reasons, an outer lenticular lens of a dual lenticular lens (facing the spectator) can also be replaced with a similar slit layer. Moreover, separately changing the curvature of lenses of both lenticular lenses of the dual lenticular lens, it is possible to adjust its distance from the projection screen, as well as the distance and the width of the optimal viewing area, preserving 3D effect. All of this is useful technological supplemental advantages of the SSE technology development.
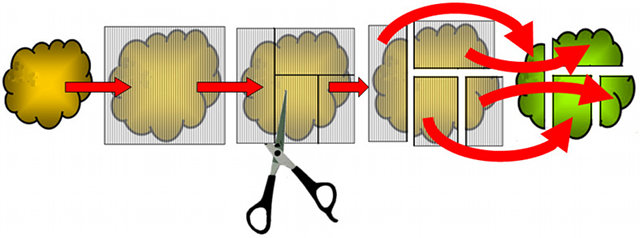
We especially should mention another feature of this technology as being fundamental, essential and direct consequence of the multi angle feature based on the Lippmann-Bonnet image and a lenticular lens: it is the additivity of 3D effect in any combination built from SSE components. We will explain this using as an example of conventional lenticular cards: if any such card would be arbitrarily cut into a number of fragments, each of them can separately produce 3D effect with respect to that portion of the chart, which is cut out of the original card (i. e. unlike holograms, it will be a local part not the whole scene). At the same time, if we take parts lenticular cards with the same lens pitch and perspective subdivision (horizontal and/or vertical) having in mind the view angles and combine them along the perimeter we will get the 3D effect of the full composition same as if the whole composition would be done from the beginning for a single lenticular lens. To illustrate said above: on the left side of the following illustration is a real scene. The next block is a printed stereo image of the scene. Then the picture is cut into arbitrary fragments. Finally, each fragment (i. e. a part of the full stereo picture) independently of others (even if some are “lost”) generates 3D stereo image, which is a part of the total scene (fragments marked in green):
The reverse sequence is also true: if separate fragments of an original scene (left picture on the bottom illustration) are subjected to the multi angle stereography using lenticular lenses of the same pitch, then by combining these stereo pictures into a single composition with the parallax in mind a viewer will see a correct 3D image of the scene (marked in green).
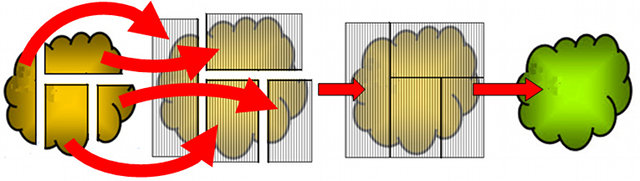
It is clear that the additivity of static stereo images is also inherited by the dynamic Lippmann-Bonnet imaging. Moreover, this, of course, refers to large arrays of lenses (a lenticular lens), which allow ignoring minor visual defects arising from the vertical (i.e. parallel to the lenses) cuts. On the conventional printed lenticular cards, these defects can be seen (zooming in using magnifying glass) on the left and right borders of the card.
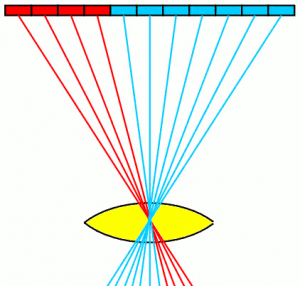In the case of combination, in which the dimensions of the lenses are substantially larger, or the Lippmann-Bonnet imagery measurements do not correspond to the lenticular lens parameters, in such case additivity is violated. The following figure shows that the separate use of “red” and “blue” parts of the Lippmann-Bonnet image would require a reconfiguration of the entire optical scheme, as the proper fragmentation of it is impossible. Just like from the parts of the same type (without additional elements) it is not possible to build an enveloping structure which would provide an integrated 3D optical effect from the combined Lippmann-Bonnet images: it suffices to note the inability even to see the related (adjacent) strips of such combined image for neighboring spectators, not to mention the complexity of synthesis of the general content.
What, generally, means the technological (and spectacular) additivity? Why this property of SSE is emphasized? Firstly, the issue consists of the following: if there is a set of similar products with the additive property, then using them as building blocks, one can create a large 3D panel of any size. It is related to consumer field, and the opportunity will immediately appreciated by advertisers, interior designers, and organizers of exhibitions, video conferencing and other specialists dealing with screen compositions.
Secondly, as important as previous is the concentration on technology needed for production of a limited range of similar products. It is related to simpler engineering studies, less intense division of labor, simpler co-operation between specialized production facilities, cheaper industrial development, rationalization of production scale and economical redundancy, simplifies training, support and production upgrades…
The third feature of SSE additivity is easily seen from the following illustrations of the static Lippmann-Bonnet image:
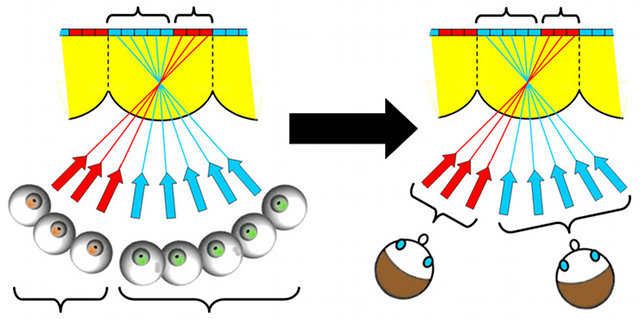
On its left side is shown how a viewer sees (using only one eye!) a striped fragment of the image located under one lens, scanning with eyes a lenticular card from edge to edge. It is clear that on the blue zone, only compactly arranged blue strips (adjacent perspectives) can be seen and the red band is visually inaccessible. However, after moving to the other end of the lenticular card, i.e. into the red zone, a viewer will see (using one eye, of course) only compactly arranged red stripes, but not a single blue strip. This means that the corresponding (blue) clusters of the Lippmann-Bonnet image can be reserved by certain content for viewer of the blue zone, and, quite independent from this, some red clusters of the same image may be loaded with autonomous content intended for the second (third, fourth, etc.) viewer located in the red viewing area.
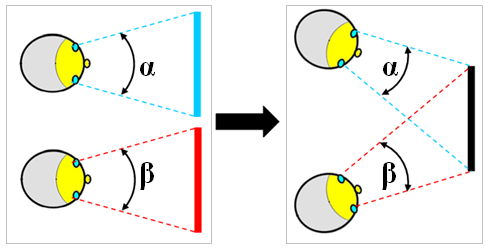
In other words, the common “passive” multiangle lenticular card demonstrates the ability of simultaneous view (preserving 3D effect) of different content for multiple viewers, which is ureachable quality in holography, but is inherent to SSE technology. A direct consequence of this (separate content for different viewers on the same screen) is a possibility to reverse this process by combining various demonstration screens into one common, as the following illustration shows (spectators with viewing angles α and β):
